The Helmholtz equation is an important differential equation. It has many applications in various fields of physics, such as optics, acoustics, electrostatics and quantum mechanics.
A lot of other related differential equations also use the methods used in solving Helmholtz equation
Index
Mathematical Background
The Equation
Expressed simply, the Helmholtz equation is written as
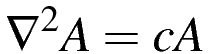
where
c is some constant
A is the function we are studying.
A common rewriting of this is
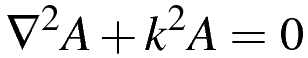
where
k has some specific applications in wave calculations.
The Terminology and Properties
The Helmholtz equation involves an operator, ⛛2, which is called the Laplacian, also written as ⛛⋅⛛.
The Laplacian takes a second-order partial derivative of the function we are considering, with respect to the spatial coordinates. Thus, the final differential equation before solving is usually a second-order differential equation.
Another property is this: the right-hand side of the equation just has the same function, multiplied by a constant. This is a special category of problems in mathematics and physics, known as Eigenvalue problems. There is rich theoretical work on eigenvalue problems and what their solutions represent.
The equation also has the property of being linear. This means that any linear combination of its solutions also gives a solution.
These properties and its nature make it a frequent occurrence in many fields of physics, some of which we shall examine now.
Applications in Physics
Wave Mechanics
The wave equation in three dimensions gives rise to the Helmholtz equation in some situations. If we attempt a solution that has space and time variables separated, we arrive at the familiar Helmholtz form with respect to the spatial part. We can use standard methods to solve this form.
Some examples in which we can see this equation are vibrating membranes (like drums), lasers, propagating sound waves, and earthquakes.
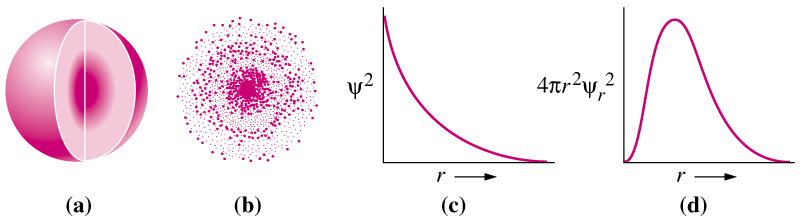
Quantum Mechanics
The cornerstone of non-relativistic quantum mechanics, Schrödinger’s equation, is an extension of the Helmholtz equation.
In this case, the right side of the equation has more than just the function A. It has an extra term, roughly corresponding to the time derivative of the function.
Again, the principles of solving are similar. The equation is first solved without considering the extra term. Then specific steps are taken to make the solution work with the additional term.
Electrostatics
A special case of this equation, the Laplace equation, turns up often in electrostatics. The Laplace equation corresponds to the case where the right-hand side is zero, so basically,
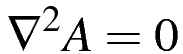
For example, we could be trying to model the electric field in a space with net zero charge.
In such cases, the process of solving is simplified. Physicists take the realistic boundary conditions and solve appropriately, separating variables in the process.
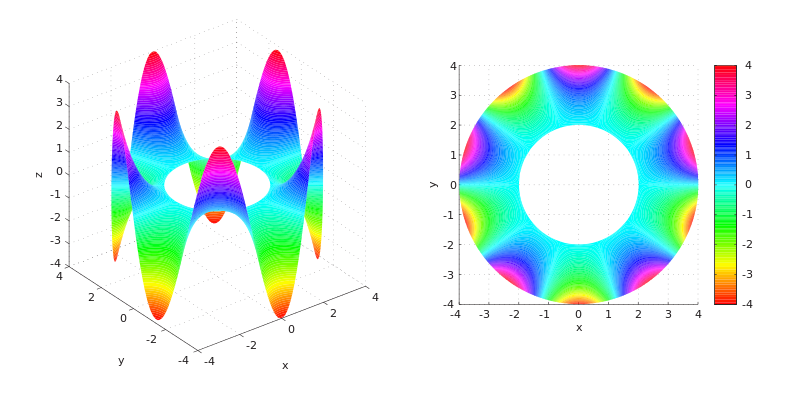
A related equation is the Poisson equation, where the right hand side is not zero, but some constant. While it is strictly not the same, the methods used in the previous case can be extended to this, with some modifications.
Conclusions
In conclusion, the Helmholtz equation is a versatile equation that turns up in many fields of physics. Its solutions have applications in diverse fields. In the study of waves, it is a very vital tool. On a more meta-level, the underlying similarity in the solutions helps us see the symmetry of nature.