Everything in this universe exists in two fundamental forms: energy or matter. Thermodynamics deals with a particular form of energy, which is heat energy. Derived from Greek it means ‘movement of heat’ and can be defined as the study of heat and its transformation to mechanical energy. Thermodynamics was developed much prior to the development of the atomic model, and hence instead of using electrons, it uses macroscopic concepts such as work, pressure, and temperature to explain thermodynamic concepts. Thermodynamics is governed by some laws which are empirical in origin or derived theoretically which we will now proceed to learn about.
Index
Zeroth Law of Thermodynamics
Prerequisites
Before we start with thermodynamics we need to understand some terms and macroscopic quantities as is required to define and understand thermodynamic systems.
A thermodynamic system exists in different ‘states’ and can move from the initial state to the final state through thermodynamic processes. The changes in the state of the system can be measured by state variables (example- pressure, temperature, volume, enthalpy, entropy, internal energy) which are independent of the type of thermodynamic process adopted.
When a hot body and cold body were put together, heat would flow from the hotter body to the colder body, until a homeostasis or as technically put, thermal equilibrium is achieved. But what is a quantitative measure to determine which body loses heat and which gains? The concept of temperature is developed. Temperature is a measure of heat, with heat flowing from a body at higher temperature to a body at lower temperature until thermal equilibrium is achieved. At thermal equilibrium the temperature of both the systems is the same. With this knowledge let’s understand what the Zeroth Law actually says.
Statement
“If two systems are in thermal equilibrium with a third system separately, they are in thermal equilibrium with each other.”
Stated by R.H. Fowler in 1931, much later than the first and second laws of thermodynamics, the law took a lower number because it was more fundamental as it defined temperature. Simply put if bodies A and C are in thermal equilibrium (at the same temperature) and body B and C are in thermal equilibrium, then body A and B are also in thermal equilibrium.
Applications
The Zeroth Law can be used to calculate the temperature of multiple objects in indirect methods as well as compare the temperatures of multiple objects. Though it seems like a rudimentary law on the surface,a law of common sense, it is important as the law develops the concept of thermal equilibrium as well as introduces the concept of temperature to measure flow of heat in a group of systems in contact.
Example
If we have a cup of boiling water at 373 K and we use a thermometer to measure the temperature, the thermometer is now in equilibrium with the water in the cup. If put in another cup of boiling water, the thermometer gives the same reading. Now when we mix both cups together, the resultant temperature will be the same (that is 373K) as they were in thermal equilibrium with the same third object (here the thermometer) and we can apply the Zeroth Law of Thermodynamics.
First Law of Thermodynamics
Prerequisites
A fundamental law that holds across the universe is the law of conservation of energy – which states that energy can neither be created nor destroyed but can be transferred from from one form to another.
Internal Energy(U)
It is the total energy of the system, which includes electronic energy, nuclear energy, chemical bond energy, potential energy and kinetic energy of the constituent molecules. It does not include the gravitational energy between the molecules. Since Internal Energy is dependent on the kinetic energy of the constituent molecules, the greater the kinetic energy, the greater the temperature, and hence greater the Internal Energy.
Pressure-Volume Work
Ideal gas systems are easiest to work with, and we derive a formula for work based on pressure-volume change.

Statement
“In a thermodynamic process involving a closed system, the increment in the internal energy is equal to the difference between the heat accumulated by the system and the work done by it.”
A closed system is one where there is no exchange of matter between the system and the surroundings, but heat exchange is allowed. The First Law of Thermodynamics is a direct result of the law of conservation of energy.
It can be understood as –
(i) If heat is provided to the system or released by the system, the internal energy rises/ falls, to maintain the fact that energy is not created but transferred from one form to another.
(ii) If work is done by the system, or work is done on the system, the internal energy falls/rises to maintain the law of conservation of energy.
The Conundrum of Conventions:
Mathematically speaking the First Law equates the change in internal energy to the heat given/lost and work done by/on the system. As per the statement that was given by Clausius, a physicist, the First Law becomes –
ΔU = q – w
where,
q is the heat given/lost
w is the work done by/on the system
ΔU is the change in internal energy
As per convention, q is positive if heat is provided to the system, and negative when released from the system. w is positive when done by the system, and negative when the work is done on the system.
But the IUPAC (International Union of Pure and Applied Chemistry) have to come and make matters confusing for us students by imposing their own conventions. As per IUPAC the First Law takes the equational form as –
ΔU = q + w
where,
q is the heat given/lost
w is the work done by/on the system
ΔU is the change in internal energy
As per convention, q is positive if heat is provided to the system, and negative when released from the system. w is positive when done on the system, and negative when the work is done by the system.
In the rest of this article, all math will be done on IUPAC conventions (because I, the author, find it easier to use 🙂 )
Applications
Change in Internal Energy can be calculated if the heat given/released by the system is known and the work done on/by the system is known. Internal Energy change is calculated for a chemical reaction by conducting the reaction in a ‘bomb calorimeter’.
The bomb calorimeter maintains a constant volume, that is, ΔV = 0 which implies that work w is equal to zero. Therefore ΔU = qv, where qv is the heat supplied/released by the system when the system is maintained at a constant volume.
Examples
Using a bomb calorimeter, any combustible substance can be burnt in pure oxygen to calculate the change in the internal energy brought about combustion. The ΔU is calculated based on formulation from the First Law of Thermodynamics.
Second Law of Thermodynamics
Prerequisites
Heat Engines
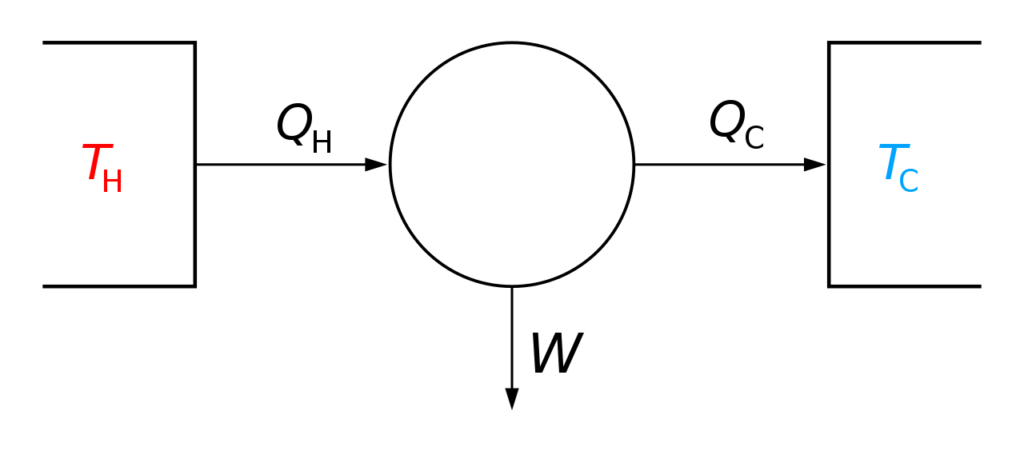
Any engine that operates on heat can be simplified to the rudimentary version that exists above. Heat (QH) is extracted from the hot reservoir at temperature TH, which the engine converts to useful work W and deposits some heat(QC) to the cold reservoir at temperature TC. Taking the example of a steam engine, the burning coal is the hot reservoir, from which QH is extracted by the engine to do work W, and some heat(QC) is deposited (or lost) to the surroundings (here, the cold reservoir) as radiation, etc.
By applying the law of conservation of energy, QH = W + QC
Statement
“No process is possible whose sole result is absorption of heat from a reservoir and the complete conversion of the heat into work.”
What this means in simple terms is QC ≠ 0 or there always has to be some heat lost(or deposited) into the cold reservoir, which is not converted into useful work.
Applications
Efficiency(η) is defined as the ratio of work output to the energy inputted. For a heat engine Efficiency(η) can be solved by the equation:
η = work ouput/energy input = W / QH = QH – Qc/ QH = 1 – Qc/QH
By the Second Law, QC ≠ 0. If we assume that the Second Law is not true, then QC takes the value of zero. Efficiency(η) becomes 1. Hence another form of stating the Second Law is that the efficiency of no machine/engine can be 1(100%). There is almost always some form of loss to the surroundings, whether through friction, heat loss, etc.
Examples
All real world engines, steam engines on trains, water heaters, heat pumps, refrigerators, everything obeys the Second Law. No engine can be perfectly efficient and there is always some form of loss of energy.
Third Law of Thermodynamics
Prerequisites
Enthalpy
Internal energy change correlates with heat transferred at constant volume. But most of the real-world chemical reactions happen in beakers where the volume is not constant. Here, the constant value is the pressure which is equal to the local atmospheric pressure at that point. We, therefore, introduce another state variable to measure change in states, called enthalpy. Enthalpy (H) is defined such that ΔH = qp. To find the relation between Enthalpy and Internal Energy, we proceed as follows –
ΔU = q + w
At constant pressure, and with w = -pΔV
ΔU = qp – pΔV ⇒ ΔU = ΔH – pΔV ⇒ ΔH = ΔU + pΔV
where,
ΔU is the change in internal energy
ΔH is the change in enthalpy
q is the heat given/released by the system
w is the work done on/by the system
p is the external pressure on the system
ΔV is the change in volume of the system
Entropy
An alternate statement to the Second Law of Thermodynamics reads, “In any spontaneous process the entropy of the universe (the system and the surroundings) always increases.”
A spontaneous process is defined as an irreversible process which has a natural tendency to occur either on its own or after proper initiation under the given set of conditions and can be reversed by some external agency only. Spontaneity hence determines the condition whether the reaction can occur or not.
Entropy is a variable introduced as a measure of the degree of randomness or disorder. The change in entropy (ΔS) is defined as the ratio of the heat absorbed by the system isothermally (at one fixed temperature) and reversibly to the temperature at which it was absorbed. Therefore,
ΔS = Qrev / T
This alternate form of the second law means that spontaneous processes result in increased entropy of the system or surroundings or both. This translated an increased disorderness of the universe.
The search of spontaneity
But how do we determine whether a reaction is spontaneous or not? Initially enthalpy was thought to be a way to figure this out. Exothermic reactions (reactions which release heat), have a negative ΔH and were seen to be spontaneous. However some endothermic reactions (reactions which absorb heat to proceed), which have a positive ΔH were also spontaneous once initiating conditions were applied. So Enthalpy is partially the answer.
What about Entropy? It was noticed that when two gases were mixed, they mixed almost homogeneously. Sugar when added to water can be seen to dissolve at the boundaries. In these real-world examples, mixing increases disorder and randomness. It seems that we have a solution to the question. Spontaneous reactions proceed with an increase in entropy. But is our answer correct?
Gibbs Free Energy
Our answer can’t be correct because we haven’t considered enthalpy, and well almost all real-world examples proceed with both a change in enthalpy and entropy. Gibbs defined a new state variable for this purpose, called Gibbs Free Energy. Gibbs-Helmholtz Reaction was derived equating a change in Gibbs Free Energy (ΔG) to the changes in enthalpy (ΔH) and entropy (ΔS). The equation reads –
ΔG = ΔH – TΔS
where,
ΔG is the change in Gibbs Free Energy
ΔH is the change in Enthalpy
ΔS is the change in entropy
T is the temperature of the system
Gibbs derived that spontaneous reactions proceed with the loss of free energy, that is, ΔG < 0 for spontaneous processes.
Let’s finally look into the Third Law of Thermodynamics.
Statement
Also called the Nerst’s Law it states that – “as the temperature of the system approaches absolute zero its entropy becomes constant or the change in entropy approaches zero”
Increasing temperature directly results in increased kinetic energy of the constituent molecules in the system, and hence an increased randomness or entropy. This means that decreasing the temperature, progressively decreases the randomness of the moving molecules, slowing them and hence bringing order. A decrease in temperature therefore decreases the entropy of the system. It should therefore be possible to progressively decrease the temperature to a point when the system is in perfect order, and has zero entropy. This implies that there exists a temperature below which no temperature value can’t possibly exist as entropy can’t have negative values.
The Third Law has thus introduced the lowest temperature theoretically possible, called absolute zero which is 0K / -273.15 oC / -460 oF.
Conclusion
With this we’ve summarised the basics behind the Laws of Thermodynamics. This article has but just scratched the surface, and there’s a lot of exciting science both to be learned and discovered in this field.
Thermodynamics is a very important field of study as it has implications from the machines we use to the internal machines that run in our bodies. It can be used to build more efficient engines and other stuff in the domains of physics. It can be used to discover new spontaneous reactions, create better chemicals, and improve methods of production of various industrial chemicals, by increasing energy efficiency.
Thermodynamic topics such as Gibbs Free Energy and Enthalpy, become important in biochemistry, especially in understanding the importance of enzymes, and why certain biochemical pathways and various catabolic and anabolic reactions take place in living systems. Thermodynamics is truly a robust and important field of study, with implications and applications everywhere.

